Vibrational dynamics of glasses remains a point of controversial discussions. The application of nuclear inelastic scattering of synchrotron radiation provides a new and consistent picture of the subject.

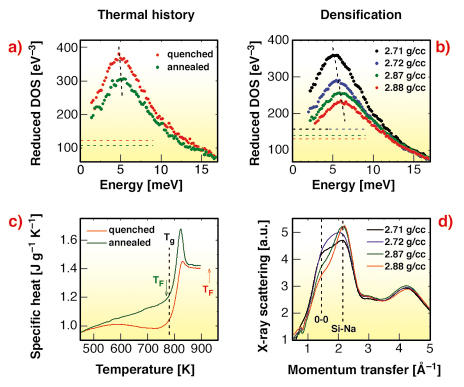
We have studied the vibrational dynamics of the iron-enriched sodium-silicate glasses with (i) different thermal history and (ii) different density. The iron-partial density of vibrational states (DOS) g(E) was measured with nuclear inelastic scattering at ID18. The reduced DOS g(E)/E2 (Figure 5) reveals an excess of vibrational states above the Debye level called the boson peak. The peak of the reduced DOS decreases in height and moves to higher energy for the annealed (Figure 5a) and densified (Figure 5b) glasses. This behaviour is usually related to a modification of the short-range or intermediate-range structure. For instance, fast quenching and slow annealing provide a glass with a structure that corresponds to “fictive” temperatures above or below the glass transition, respectively. The change of the structure with densification is usually related to the evolution of the first sharp diffraction peak in the static structure factor.

Fig. 5: Reduced density of vibrational states of glasses with (a) different thermal history and (b) different density. The horizontal lines show the calculated Debye levels. The black dashed lines emphasise the shift in the peak position. (c) Calorimetry data for the glasses with different thermal history. The vertical dashed line shows the glass transition temperature. The arrows indicate the corresponding fictive temperatures TF. (d) X-ray scattering data for the densified glasses. The dashed lines indicate the positions corresponding to the characteristic oxygen-oxygen and silicon-sodium atoms distances.

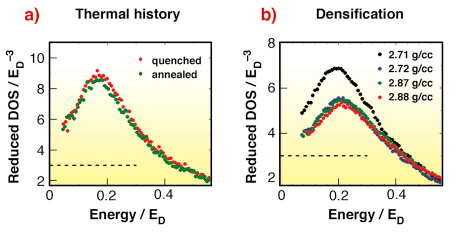
The properties of the glasses were characterised with various techniques. Calorimetry confirmed high efficiency of quenching (Figure 5c): the calculated fictive temperatures for the quenched and annealed glass are 19% above and 2% below the glass transition, respectively. However, Mössbauer spectroscopy and X-ray scattering did not reveal any relevant effect of quenching on the microscopic or intermediate-range structure. On the other hand, quenching affected the macroscopic parameters: the density and the sound velocity for the quenched glass are smaller than for the annealed one. The changes in density and sound velocity suggest that the observed transformation of the reduced DOS (Figure 5a) could be described by the transformation of the elastic medium. In order to evaluate the role of elastic medium, the densities of states were rescaled in Debye energy units and renormalised. After this correction for the macroscopic parameters, the reduced densities of states become identical (Figure 6a).

Fig. 6: Reduced density of vibrational states of glasses with (a) different thermal history and (b) different density after rescaling the energy axes in Debye energy units and the renormalisation of the DOS area. The horizontal lines show the Debye levels.

For the densified samples, the transformation of the glass follows a slightly more complicated path. On the first step of densification, the macroscopic parameters do not change much: the density shows only a slight increase from 2.71 to 2.72 g/cc. The sound velocity does not change significantly either. On the other hand, Mössbauer spectroscopy reveals that the initial step is accompanied by a notable transformation of the local environment of the iron atoms, which is much less pronounced upon further densification. The intermediate-range order seems to change continuously with densification: X-ray scattering reveals monotonous transformation of the first sharp diffraction peak (Figure 5d). After correction for the transformation of the elastic medium, the reduced densities of states for all densified glasses except for the initial one become identical (Figure 6b). Thus, the change of the microscopic structure on the initial step of densification causes changes in the density of vibrational states which cannot be accounted for by corresponding transformation of the elastic medium. On the other hand, if the microscopic structure does not change, the transformation of the DOS is entirely described by the transformation of the elastic medium.

The results suggest that for a glass with a given microscopic structure the transformation of atomic vibrations is sufficiently described by the corresponding transformation of the elastic medium. Other parameters such as the fictive temperature or the shape of the first sharp diffraction peak do not appear to be correlated to the density of vibrational states. Furthermore, neither density nor thermal history change the boson peak defined as the excess of the vibrational states above the Debye level: the changes in the number of states are compensated by the changes of the Debye level.

 

 

Principal Publications and Authors

A. Monaco (a), A.I. Chumakov (a), G. Monaco (a), W.A. Crichton (a), R. Rüffer (a), D. Fioretto (b), L. Comez (b), Y.-Z. Yue (c), A. Meyer (d), and J. Korecki (e,f), Phys. Rev. Lett. 96, 205502 (2006), Phys. Rev. Lett. 97, 135501 (2006).
(a) ESRF
(b) Univ. Perugia (Italy)
(c) Univ. Aalborg (Denmark)
(d) TUM Physik-E13, Garching (Germany)
(e) AGH Univ. of Science and Technology, Kraków (Poland)
(f) Institute of Catalysis and Surface Chemistry, Polish Academy of Science, Krakow (Poland)