Towards full polarisation control in resonant inelastic soft X-ray scattering

Inelastic X-ray scattering yields information about materials through the transfer of energy and momentum from the photons to the sample. Energy and momentum lost by the X-rays define the excited state of the system at the end of the scattering process. This conceptual scheme is widely used for a variety of energy loss experiments that use electrons, neutrons, optical photons or X-rays, with the purpose of studying the dynamical, electronic and magnetic properties of condensed matter. The peculiarity of RIXS (resonant inelastic X-ray scattering) is that the incident photon has an energy corresponding to an internal transition energy (i.e. an absorption resonance). RIXS is of particular interest in the soft X-ray regime, where the 2p→3d transition (L_2,3 edge) is ideally suited for the study of 3d transition metal compounds, such as high T_c cuprate superconductors, colossal magneto-resistance manganites and several other fascinating oxides. For example, it is possible to use RIXS at the L edges to detect magnetic excitations (dispersing spin waves) and d to d orbital excitations usually forbidden by electric dipole transition selection rules.

Since the resonances in RIXS spectra are the fingerprints of the chemical elements and their ionisation states, RIXS experiments are chemically specific and sensitive to the environment of the excited ions. The challenge is thus to fully exploit this intrinsic selectivity. Together with better energy resolution, higher selectivity can arise from the knowledge of the polarisation state of the photons, both before and after the scattering event. In fact, the polarisation of the scattered photon is specifically modified depending on the character of the excitation created in the sample. For the incident beam, modern undulator sources have already allowed full polarisation control for several years. However, until now, the polarisation of scattered photons had never been measured in RIXS experiments in the soft X-ray range due to technical difficulties, contrary to the hard X-ray regime where the experimental feasibility has already been proven [1].

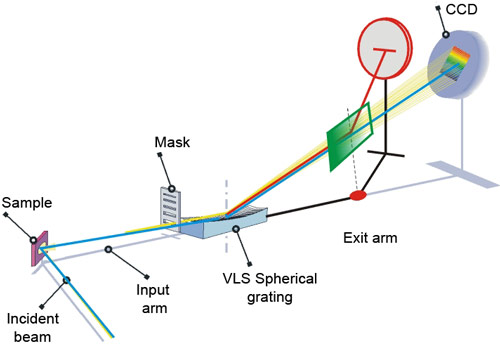
RIXS with linear polarisation analysis in the soft X-ray range has been achieved for the first time at beamline ID08 by modifying the existing spectrometer, called AXES. The initial prototype had been designed to work at the Cu L₃ edge. The sensitivity to linear polarisation is provided by a W/B₄C multilayer mirror operated at a 40 degree total deflection angle (not far from the ideal value dictated by the competition of polarisation sensitivity and intensity), so that the reflectivity of the σ component is larger than that of the π component (from Fresnel laws, R_π/R_σ ≈ cos²2θ).  The optical layout is given in Figure 1. The multilayer is installed in the output arm i.e. past the grating analyser. Note that the combined resolving power E/ΔE ≈ 3000 is identical with and without the polarimeter.

Figure 1. Optical layout of the AXES spectrometer with the multilayer mirror mounted between the grating analyser and the CCD detector. The “traditional” optical path is in blue. When the multilayer is inserted, the beam gets deflected by 40 degrees (red line) and the detector is moved accordingly. The multilayer rotates around the axis normal to the output arm..

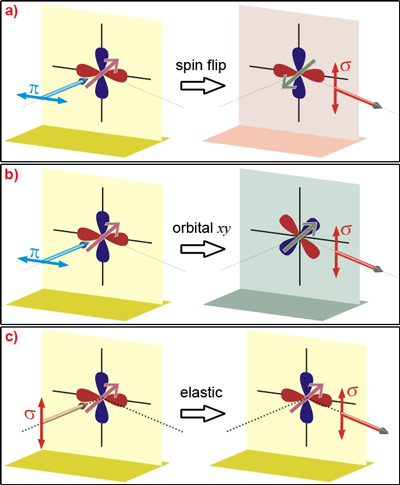
The first experiment using the new linear polarisation analysis was for layered cuprates: Cu L₃ RIXS of the infinite layer CaCuO₂. Previously, during RIXS at the Cu L₃ threshold (2p_3/2 to 3d) of layered cuprates (parent and superconducting compounds), it was demonstrated that the scattering of photons featuring π polarisation at approximately normal incidence leads, with high probability, to a spin flip, i.e. to a magnon excitation [2, 3]. Theoretically, a spin flip must be accompanied by a rotation of the linear polarisation vector of the scattered photon (see Figure 2a) [4]. The dd excitation corresponding to a transition from the ground state with a 3d hole having x²-y² symmetry to an excited state of xy symmetry (Figure 2b) would be similar [5]. However, an elastic scattering process preserves the photon polarisation (Figure 2c).

Figure 2. Schematic representation of selected RIXS processes in Cu L3 edge RIXS. a) For a spin–flip process, ultimately leading to single magnon excitation, the incident π polarisation gets rotated and pure σ polarisation is present in the scattered beam. b) A pure x2-y2 to xy orbital excitation is similar. c) The σ polarisation is preserved for elastic scattering.

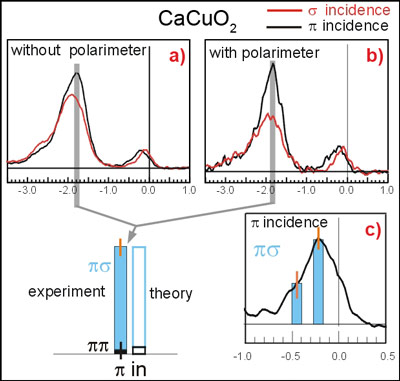
By using both σ and π incident polarisation, and by collecting polarisation dependent spectra, four spectra were obtained, allowing the linear polarisation properties of the scattered photons to be determined. For undoped CaCuO₂ the raw data are shown in Figure 3a-b. The decomposition of the spectrum with π incidence, integrated over the two grey regions, is represented by the bars in the lower panels. Clearly the main peak (1.9 eV) is dominated by the crossed polarisation channel πσ. The theoretical weights of the πσ and ππ components (bars without filling) obtained from a single ion model for the x²-y² to xy excitation (Figure 1c) agree well with the experimental finding [5]. This new approach is also important for the study of low energy magnetic excitations, as shown in Figure 3c. Within the statistical uncertainty, the ππ component vanishes not only at the main single-magnon peak at ~0.2 eV, as expected from theory (Figure 1b), but also at larger energy transfer, indicating that higher order terms also belong to the πσ crossed polarisation channel. This discovery is of great help for the interpretation of RIXS intensities in magnon spectroscopy.

Figure 3. RIXS spectra of CaCuO2 at q ≈ 0.25 r.l.u. in the (1,0) direction, using σ (red) and π (black) incident polarisation. The spectra were measured a) without the polarimeter in the scattered beam, and b) with the polarimeter in the scattered beam. By combining the four spectra and using the known polarisation transmission factor, the 2 components of the scattered beam can be evaluated for π incident polarisation at the main dd excitation peak and at the magnetic peak. For the dd peak the experimental result is compared to the theoretical expectation for the x2-y2 to xy excitation.

Authors
L. Braicovich (a), M. Moretti Sala (a,b), M. Minola (a), G. Trezzi (a), A. Di Natale (a), G. Ghiringhelli (a),  F. Yakhou-Harris (b), C. Morawe (b), L. Eybert (b), A. Fondacaro (b), N.B. Brookes (b).
(a) Politecnico di Milano (Italy)
(b) ESRF

References
[1] K. Ishii et al., Phys. Rev B 83, 241101 (2011).
[2] L. Braicovich et al., Phys. Rev Lett. 104, 077002 (2010); Phys. Rev B 81 174533 (2010).
[3] M. Le Tacon et al., Nature Physics 2041 (2011).
[4] L. Ament et al., Phys. Rev. Lett. 103 117003 (2009).
[5] M. Moretti Sala et al., New J. Phys. 13 043026 (2011).

Top image: Polarimetric soft X-ray RIXS of cuprates.