- Home
- News
- Spotlight on Science
- 3-Dimensional Coherent...
3-Dimensional Coherent X-ray Diffraction on a single Au Crystal of Micrometre Size
20-07-2004
The use of coherence opens the way to 3D imaging of micrometric crystals with nanometric resolution.
The phase problem can be solved by the over-sampling of a diffraction pattern using a coherent beam. Therefore, 3D real space density distributions can be obtained without the need for a priori hypothesis [1]. Application of this technique to the X-ray regime is still a challenge [2] and was recently performed on the ID01 beamline.
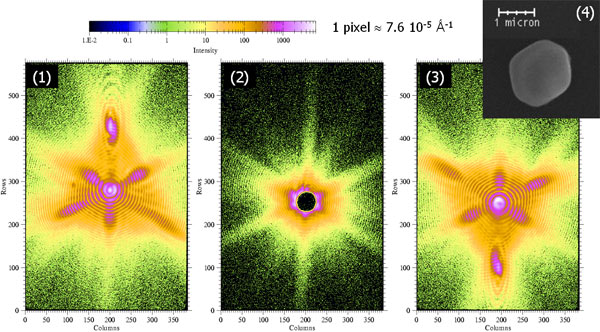
Figures 1 to 3 show the results of a coherent X-ray diffraction experiment on a single Au crystal of 1.5 µm size, obtained by accumulating 1000 frames measured by a direct illumination CCD [3]. Figure 4 shows a SEM picture of the facets of the sample crystal. The coherence length at ID01 allows the observation of tens of interference fringes (resulting from finite size effects) in the experimentally chosen (111) diffraction pattern.
In the absence of strain, a 3D centro-symmetric Bragg spot is expected. This property is visible on the pattern measured at the centre of the Bragg spot (Figure 2). Additionally, a centro-symmetry operation allows the transformation of Figure 1 into Figure 3, corresponding to cuts through the spot for an angular offset of ±0.08° from the Bragg maximum. The intense streaks result from the crystal facets.
The full 3D-pattern will be used for the inversion in order to obtain a real space image of the crystal. This experiment opens the way to coherent diffraction measurements on nano crystals and quantum dots on the ID01 beamline.
Authors
V. Chamard, F. Livet, K. Ludwig, F. Bley, M. de Boissieu and I. K. Robinson
References
[1] J. R. Fienup, Appl. Opt. 21, 2758 (1982), R. W. Gerchberg and W. O. Saxton, Optik (Stuttgart) 35, 237 (1972).
[2] I. K. Robinson, et al., Phys. Rev. Lett. 87 195505 (2001).
[3] F. Livet, et al., Nucl. Instr. Meth. A 451, 596 (2000).