- Home
- Users & Science
- Find a beamline
- Structure of materials
- ID19 - Microtomography beamline
- ID19 Techniques
- Diffraction Topography
- X-ray diffraction imaging of electric field induced modifications in crystals used in non-linear optics applications
X-ray diffraction imaging of electric field induced modifications in crystals used in non-linear optics applications
Petra Pernot
Some highlights of diffraction imaging investigations of LiNbO3, a-LiIO3, KTiOPO4 (KTP) and KAsOPO4 (KTA) crystals performed at ID19 beamline is presented. They have a variety of significant technological applications due to their piezoelectric, elastic and non-linear optical properties. In particular, the knowledge of the behaviour of these compound under an applied electric field is important for the applications.
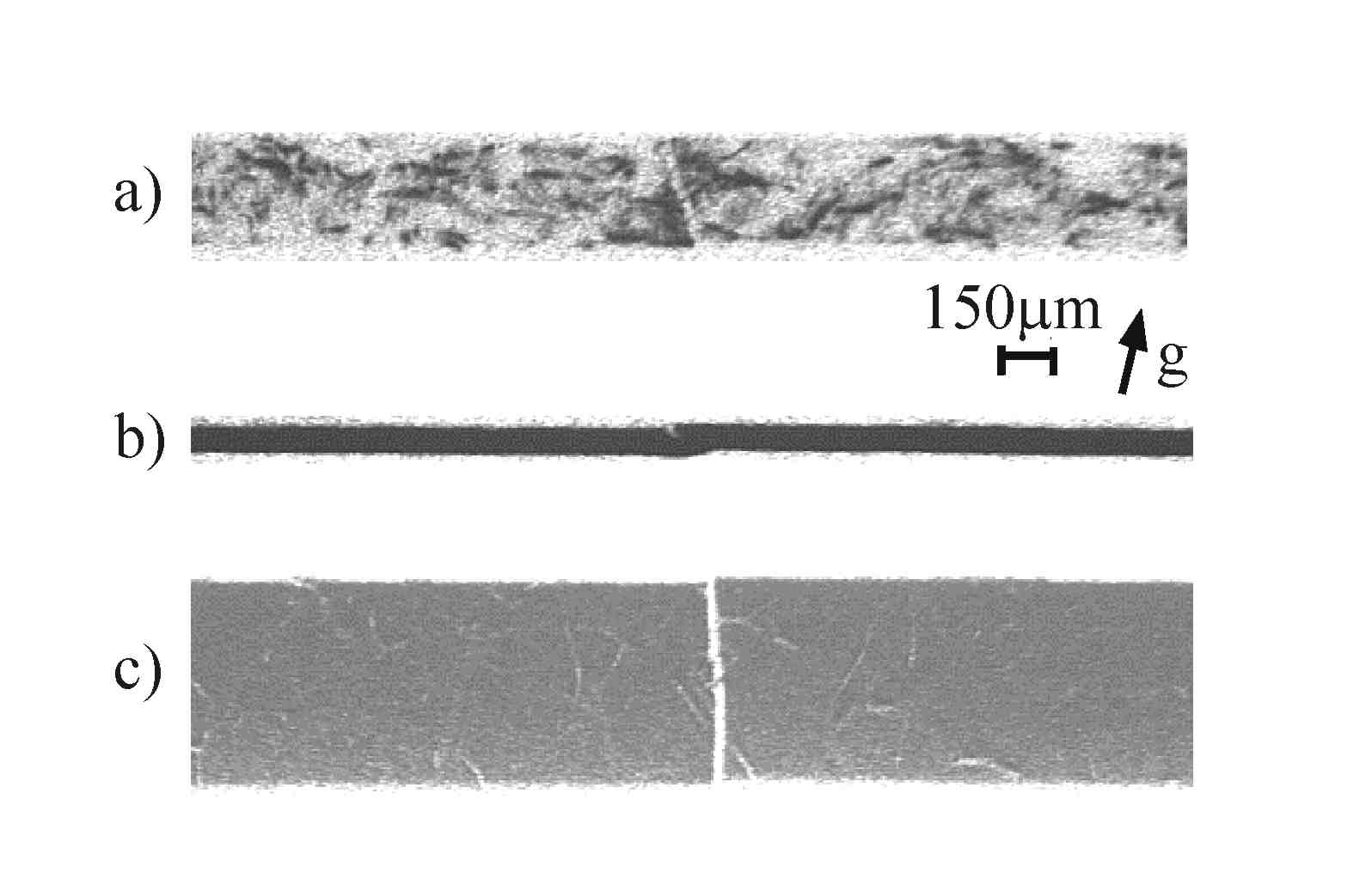
A) The diffracted intensity of LiNbO3 samples increases in the area of the sample under the electrodes and an expansion, or contraction, of the image of the bulk occurs according to the orientation of the applied field, for a given lattice plane, see fig.1. This focusing effect is associated with a curvature of the lattice planes under an applied field. Experimental evidence (see the result of the etching on fig 2) and a theoretical analysis based on the equations of the static electro-mechanical field in a piezoelectric medium, allow us to explain the reversed domain-related origin of the observed curvature [1].
 |
 |
| Figure 1 | Figure 2 |
Fig.1: Section topographs of the Y-cut sample, -1017 reflection, l = 0.34Å, where g is projection of the diffraction vector on the film. a) E =0, b) E =+3.2´ 10 6Vm -1, c) E = -3.2´ 10 6Vm -1.
Fig.2: Reflected light micrographs of the surface areas near the former electrode edges of the Y-cut sample after etching.
Fig.2: Reflected light micrographs of the surface areas near the former electrode edges of the Y-cut sample after etching.
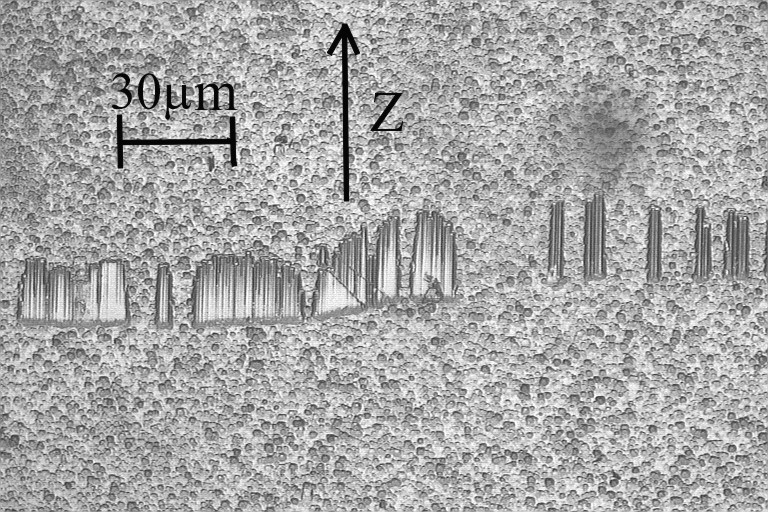
B) Lithium niobate, KTP and KTA crystals can achieve optical second-harmonic generation with enhanced efficiency when a periodic reversal of the sign of the non-linear optical coefficient d33 occurs in the phase matching period. This periodically inverted domain structure can be produced by applying an external electric field using patterned electrodes. Fresnel and Bragg diffraction were used simultaneously to visualise these ferroelectric domains within the bulk. The wavefront of the Bragg-diffracted X-ray beam is split by the phase difference between the structure factors of adjacent domains. This shift is measured through quantitative analysis of the image contrast as a function of propagation distance [2]. Fig.3 shows the experimental and simulated images as a function of defocusing distance D of the 006 reflection of a periodically poled (period 14.8mm) LiNbO3 sample. The calculated and measured phase shifts are in very good agreement, being both equal to 140°. The internal distribution of domains can be visualised using section topography technique. Fig.4 represents section topographs at various sample-to-detector distances of a periodically poled KTA crystal (period 39mm). From the numerical analysis of the intensity variations after free space propagation, it was suggested that the atom As(1) most likely acts as a linking/pivot atom for connecting inversion domains across (100) domain walls [3]. This approach allows the investigation of any feature of a crystal which introduces a distortion of the phase of the Bragg diffracted wave. To the best of our knowledge, there is the only technique which allows one to distinguish between different schemes for matching domains across a boundary and for revealing the position of the inversion centre. A similar investigation applied to periodically poled KTP crystals and the low temperature study are under way. The internal distribution of domains can be investigated even at small sample-to-detector distances if an electric field is applied, due to additional distortions produced via the piezo-electric effect [4].
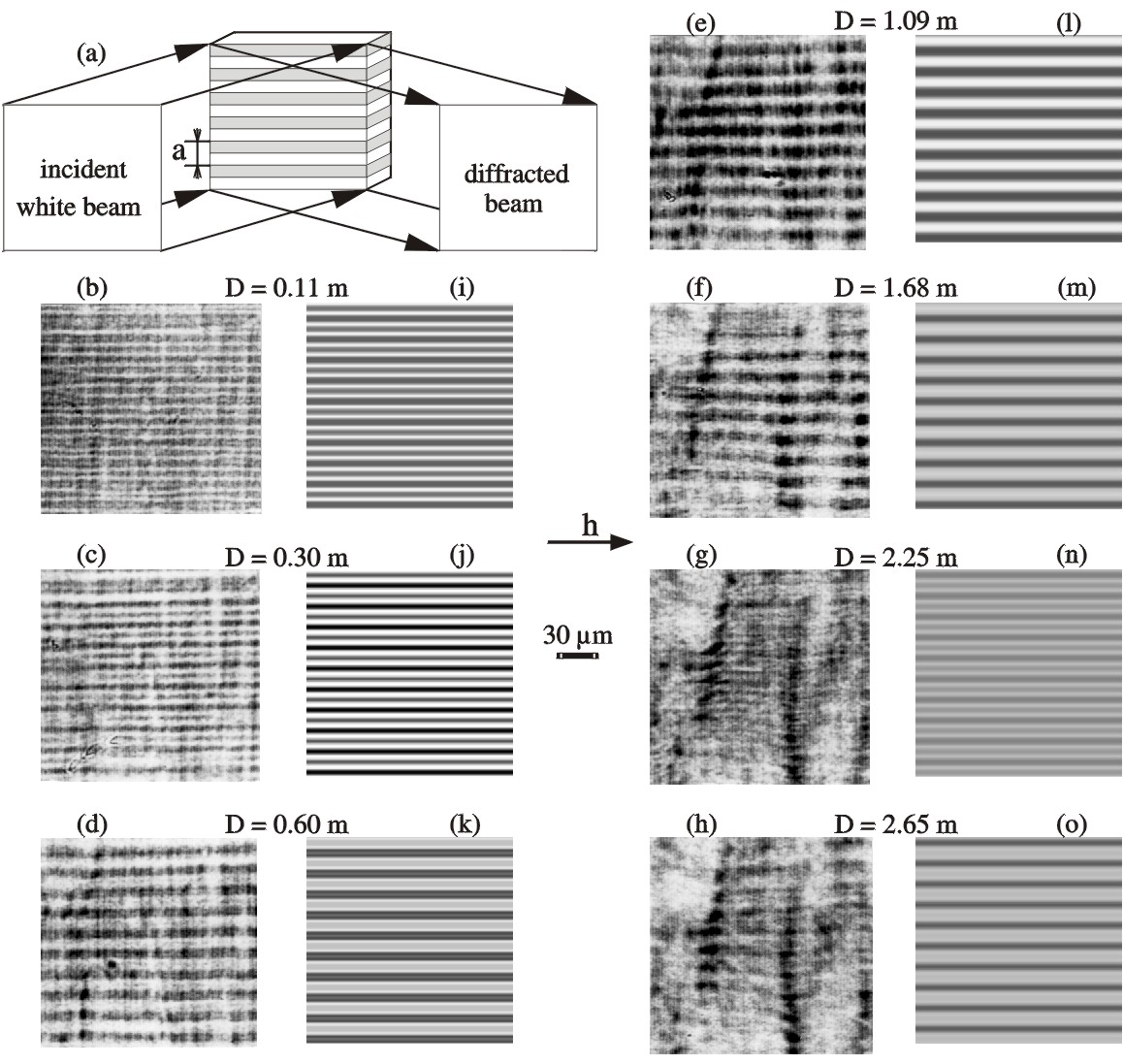
Fig.3: Experimental and simulated images of 006 reflection of a lithium niobate sample, l= 1Å
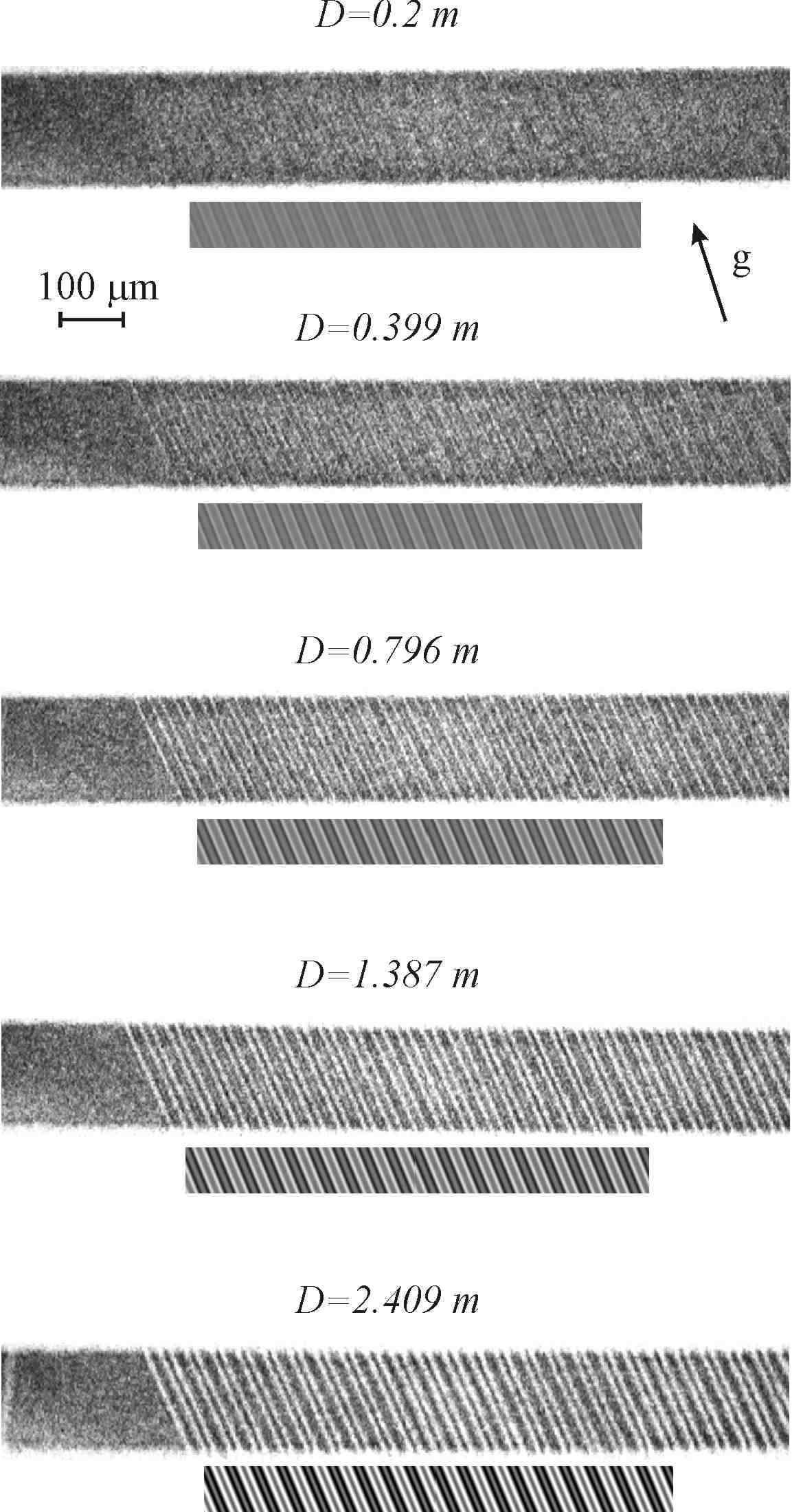
Fig.4: White beam section topographs of a KTA sample as a function of the defocusing distance D using the 1  2 reflection, l = 0.255Å, The contrast simulations are presented below the experimental images considering Dj = -21.1°(atom As(1) supposed to be the origin)
2 reflection, l = 0.255Å, The contrast simulations are presented below the experimental images considering Dj = -21.1°(atom As(1) supposed to be the origin)
C) KTP and a-LiIO3 single crystals have been studied under a dc electric field applied along the polar axis. Earlier experiments have revealed, that the X-ray Bragg diffracted intensity is enhanced by a factor up to ten in the low absorption case. This enhancement is mainly produced by line defects parallel to the c axis. They are observed on the topographs recorded using the 00l planes, fig.5. The effect is not present on the hk0 topographs and disappear when removing the electric field [5]. Tentative explanations have been proposed to account for these line defects, but none of them explain the whole set of experimental facts. The new facts are that these lines constitute a highly temporary effect, which propagates from the anode to the cathode, reaches a maximum and decays to progressively disappear, the crystal being still subjected to the applied electric field. The phenomenon remains the same when the temperature is changed, but its typical time is dramatically increased when the temperature is decreased. These facts suggest that the line defects are related to inhomogeneous ionic conduction through 1) the potassium ion motion via irregular sites and 2) the inhomogeneities of the electrodes. An appropriate model explaining all the experimental evidences is under development [6].
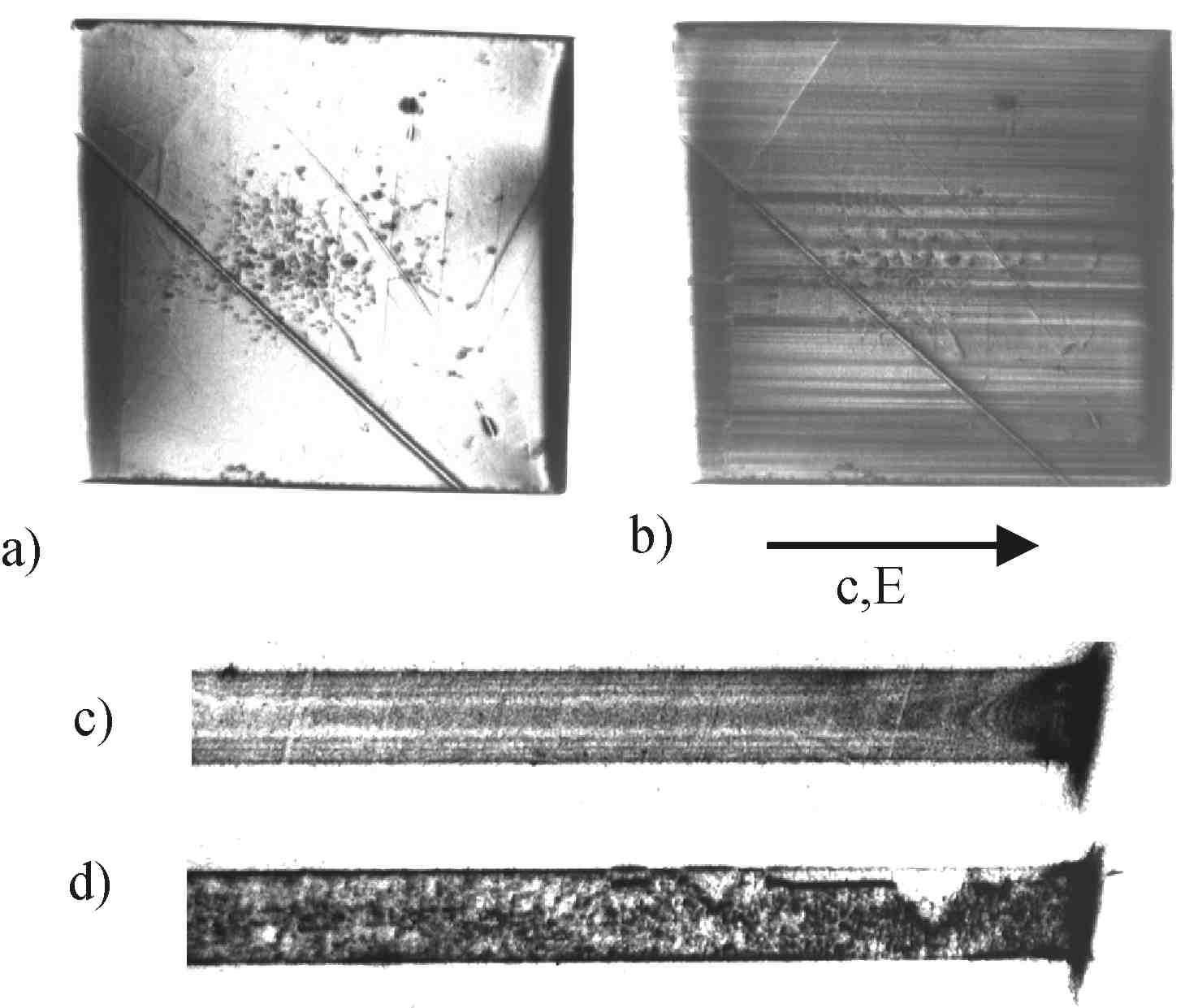
Fig.5: Projection (a,b) and section (c,d) topographs of a b-plate KTP sample without (a,c) and under dc electric field applied along the c-axis equal to 60V/cm. Dimensions of the sample are 10x10x1mm 3. Slit was set perpendicularly to the c-axis.
This kind of imaging investigations benefits greatly from the properties of X-ray beam available at ID19 such as coherence, homogeneity, high flux and high energy. In the future the new (bigger) experimental hutch will permit to push further the coherence-related experiments.
[1] P. Pernot-Rejmánková, W. Laprus and J. Baruchel, Eur. Phys. J. AP 8 (1999) 225
[2] P. Rejmánková-Pernot, P. Cloetens, J. Baruchel, J.P. Guigay and P.Moretti, Phys. Rev. Let. 81 (1998) 3435
[3] P. Pernot-Rejmánková, P.A. Thomas, P. Cloetens, F. Lorut , J. Baruchel, Z.W. Hu, P. Urenski and G. Rosenman, J. Appl. Cryst. 33 (2000) 1149
[4] P. Rejmánková, J. Baruchel, P. Moretti, M. Arbore, M. Fejer and G. Foulon, J. Appl. Cryst. 31 (1998) 106
[5] P. Rejmánková, J. Baruchel and J. Kulda, Phil. Mag. B 75 (1997) 871
[6] F. Lorut, PhD Thesis



