- Home
- News
- Spotlight on Science
- Stellar representation...
Stellar representation of quantum dynamics
22-06-2012
A novel geometric description of quantum dynamics is given by Majorana’s stellar representation of quantum spin as points on a sphere.
Eighty years ago, the famous Italian physicist Ettore Majorana (who later mysteriously disappeared at the age of 32) invented an elegant, yet still largely ignored, representation of finite quantum systems, in which a pure quantum state of a spin-J system is viewed as a constellation of 2J stars on the celestial sphere [1].
Majorana’s stellar representation, being purely geometric, allows quantum mechanics to be reconciled with the concept of “trajectory” which is the hallmark of classical physics and the basis of our physical intuition in the classical realm, but is lost in the usual Schrödinger representation (based upon a wavefunction delocalised over the whole space) or the Heisenberg representation (based upon matrices) of quantum mechanics: in Majorana’s representation, a quantum trajectory of a spin-J system is precisely described as the trajectory of 2J points on a sphere, which is the phase space of our quantum spin.
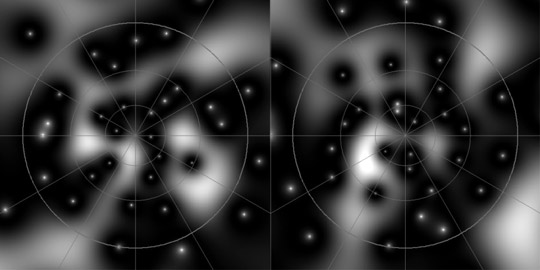
However, in order to be able to use this representation, one needs to know how to express the expectation value of the physical observables (dipole moment, quadrupole and higher multipole moments, energy, etc.) in terms of the Majorana stars. This is achieved for the first time in the present work, via the development of a novel diagramatic method, allowing the expression of the value of any observable in terms of the Majorana stars. This is realised by mapping the quantum state of a spin-J system onto the thermodynamic partition of a (fictitious) classical gas on the sphere, subject to the Coulomb repulsion of the 2J Majorana stars. This is illustrated in Figure 1.
Besides the calculation of the energy and its derivatives (yielding the “forces” acting on the system), it is also necessary to know the so-called “symplectic structure” (which determines how the system evolves in response to forces). In quantum mechanics, the symplectic structure is given by the “geometric phase”, which plays a central role in quantum mechanics: upon cyclic motion of the stars on the sphere, the quantum system acquires a phase shift, called geometric phase, discovered in the 1980's by Berry, Aharonov and Anandan [2,3]; the related “geometric curvature tensor” (infinitesimal version of the geometric phase) determines how the system evolves when subjected to external forces. In this work, it is also shown that the fictitious classical gas surrounding the Majorana stars and interacting with them actually consists of so-called “Dirac strings” (magnetic field filaments containing exactly one quantum of magnetic flux). The geometrical phase of the Majorana stars corresponds to the Aharonov-Bohm phase(*) acquired during their motion among the gas of Dirac strings. Explicit formulas for the calculation of the geometric curvature tensor, as well as for the quantum metric tensor (which determines the outcome of quantum measurements), of spin systems in terms of the Majorana stars are given for the first time.
Finally, using the above mentioned concepts and results, the equation of evolution for quantum spin systems is obtained, in terms of the majorana stars. This novel, purely geometric, description of quantum dynamics, which constitutes the quantum mechanical counterpart of the celebrated Landau-Lifshitz equation [4] of classical spin systems is expected to shed new light on various systems such as molecular magnets, spin nematics, quantum atomic gases and quantum Hall systems.
(*) The Aharonov-Bohm effect is a topological quantum effect in which charged particles get "aware" of the magnetic field present in regions they can go around but never penetrate, by acquiring a phase proportional to the enclosed magnetic flux. This Aharonov-Bohm phase can be revealed by suitable interference experiments, and has many important physical consequences.
Principal publication and authors
Quantum Geometric Phase in Majorana’s Stellar Representation: Mapping onto a Many-Body Aharonov-Bohm Phase, P. Bruno (a), Phys. Rev. Lett. 108, 240402 (2012).
See also the accompanying Viewpoint : A Quantum Constellation, Q. Niu, Physics 5, 65 (2012).
(a) ESRF
References
[1] E. Majorana, Nuovo Cimento 9, 43 (1932).
[2] M.V. Berry, Proc. R. Soc. A 392, 45 (1984).
[3] Y. Aharonov and J. Anandan, Phys. Rev. Lett. 58, 1593 (1987).
[4] L.D. Landau and E.M. Lifshitz, Phys. Z. Sowjetunion 8, 153 (1935).
Top image: Part of a spherical representation of Majorana stars for a quantum state with spin J=25.