Electric field induced selective disordering of lamellar block copolymers

Block copolymers consist of two or more chemically distinct polymer segments. They readily self-assemble into a wide range of periodic structures, which exhibit characteristic feature sizes on nanoscopic length scales (10 – 100 nm).

Generally, the spontaneous ordering of soft matter is driven by weak noncovalent interactions between its molecular constituents. While these weak interactions drive self-organisation over mesoscopic length scales, they also permit high densities of structural imperfections. Such defects, however, severely limit the spatial coherence of self-organised soft matter. Consequently, a wide range of techniques are being explored to direct the formation of highly ordered structures that exhibit both translational and orientational order on macroscopic length scales.

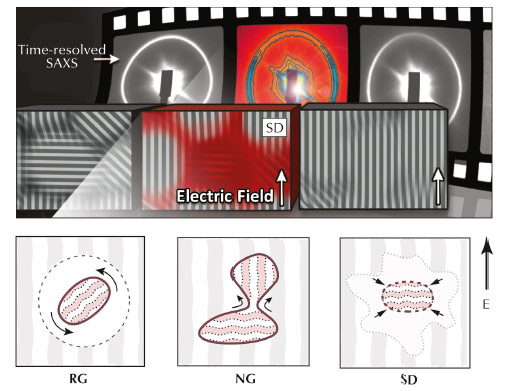
At beamline ID02 we studied the effect of an external electric field on the orientational and translational order of block copolymer lamellae close to the order-disorder transition (ODT). Typically, lamellar alignment proceeds via two distinct alignment mechanisms: rotation of grains (RG) and nucleation and growth (NG) [1]. The main selection criterion is the segregation strength between chemically unlike components. Strongly segregated block copolymers align through the migration and annihilation of grain boundary defects, which enables the rotation of entire lamellar grains (RG). Contrary to this, less strongly segregated lamellae may align through NG. Here, perpendicularly aligned lamellae are destabilised and field-induced undulations nucleate the formation of parallel-aligned lamellae (Figure 76).

Fig. 76: Time-resolved SAXS data obtained from a lamellar block copolymer solution close to ODT upon the inception of an electric field indicating the selective disordering (SD) of ill-aligned lamellae. The images in the front illustrate the situation in real-space (top). Electric field-induced lamellar alignment typically proceeds via either rotation of grains (RG) or nucleation and growth (NG) for strongly and moderately segregated lamellae, respectively. SD occurs in proximity to the ordering transition (bottom).

Yet another mechanism can be envisaged close to ODT, that is, for weak segregation. Here, ill-aligned lamellae selectively disintegrate in the presence of an electric field, forming patches of a disordered melt, which may spawn parallel-aligned lamellae when the applied field ceases (Figure 76). Selective disordering (SD) relies on a small difference between the order-disorder transition temperatures (T_ODT) between ill-aligned lamellae [2], which has long been seen to be too marginal to be of any practical importance. However, recent computer simulations suggest that SD might yet be within experimental reach [3].

The electric field-induced alignment of a lamellar phase generally gives two populations of lamellar orientations: a major population that is aligned parallel to the electric field, and a minor population that remains perpendicularly aligned where the lamellar interface is oriented parallel to the capacitor’s electrodes. An X-ray area detector conveniently allowed us to monitor the evolution of translational order for all lamellar orientations, simultaneously. To quantify its degree, we used the transverse correlation length ξ between distinct lamellae, that is, the length scale over which lamellar layers are spatially correlated.

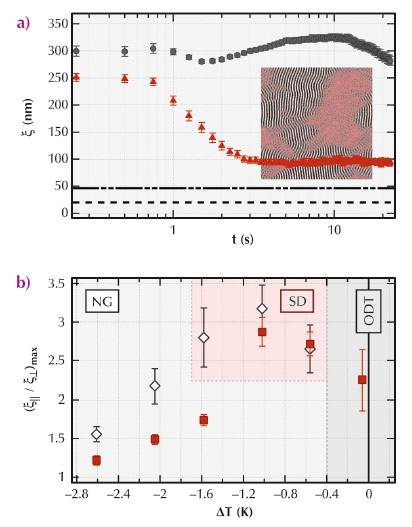
Fig. 77: Time-evolution of the translational correlation length ξ upon inception of an electric field of 1 kV/mm at ΔT = -1K below TODT (a). The degree of the asymmetric response of ξ for parallel and perpendicularly aligned lamellae delineates a small region below ODT, where SD becomes prevalent (b).

Figure 77a depicts the time evolution of ξ upon inception of an electric field of 1 kV/mm in proximity to ODT. Data are shown for lamellae, which are aligned parallel and perpendicular to the applied field. Notably, ξ drops substantially for perpendicularly aligned lamellae, while ξ remains mostly unaffected for lamellae that are already oriented parallel to the field direction. Indeed, ξ for perpendicularly aligned lamellae approaches values that are usually found above ODT in the disordered phase. That is, any long-range transitional order is lost.

Examining the degree to which lamellae lose translational order depending on orientation and distance from ODT unveiled a narrow region in proximity to ODT where SD becomes significant (Figure 77b). In this region, ill-aligned lamellae dissolve, while parallel-aligned lamellae remain mostly unaffected. This mechanism was further sustained through dynamic density-functional theory (DDFT) simulations, which illustrate the situation in real space (Figure 77a inset). Our findings suggest that moderate electric fields may well be sufficient to alleviate grain boundary defects even close to the ordering transition.

Indeed, by examining the evolution of the translational order for all known orientation mechanisms, we found that long-range translational order may well be improved through the application of an electric field for either strongly and weakly segregated block copolymer lamellae. However, we find no improvements on the long-range translational order when alignment proceeds through NG.

Principal publication and authors

M. Ruppel (a), C.W. Pester (b), K.M. Langner (c), G.J.A. Sevink (c), H.G. Schoberth (b), K. Schmidt (d), V.S. Urban (a), J.W. Mays (a) and A. Böker (b), ACS Nano 7, 3854 (2013).

(a) Oak Ridge National Laboratory (ORNL) (USA)

(b) RWTH Aachen University (Germany)

(c) Leiden University (The Netherlands)

(d) SLAC National Accelerator Laboratory (USA)

References

[1] A. Böker, H. Elbs, H. Hänsel, A. Knoll, S. Ludwigs, H. Zettl, V. Urban, V. Abetz, A.H.E. Müller and G. Krausch, Phys. Rev. Lett. 89, 135502 (2002).

[2] K. Amundson, E. Helfand, X.N. Quan, S.D. Hudson and S.D. Smith, Macromolecules 27, 6559 (1994).

[3] G.J.A. Sevink, M. Pinna, K.M. Langner and A.V. Zvelindovsky, Soft Matter 7, 5161 (2011).