Aharonov-Bohm interferences from local deformations in graphene

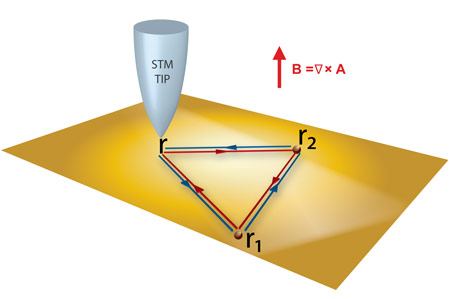
The Aharonov-Bohm effect is one of the hallmarks of quantum physics, widely established as the textbook illustration to explain the physical content of the electromagnetic gauge fields [3]. In the context of quantum transport in meso- and nanoscopic devices, in particular, it was found that the Aharonov-Bohm effect gives rise to a peculiar magnetoresistance behaviour due to the specific way in which it touches on the phenomenon of weak localisation [4]. More recently, a scanning-tunnelling-microscopy (STM) setup has been put forward to visualise directly the Aharonov-Bohm effect through oscillations in the local density of states (LDOS) [5]. The STM is a very convenient tool for studying the properties of the 2D electron gases that form at the close-packed surfaces of noble metals such as Cu(111) or Ag(111), where the proposed Aharonov-Bohm setup can easily be implemented. The main idea is to replace the open electron paths of the standard Aharonov-Bohm interferometers by the closed loops depicted in Figure 1. Thus, in the presence of a time-reversal symmetry-breaking gauge field, electrons scattering along these loops pick up different phases depending on whether the scattering is clockwise or anticlockwise. This further affects the interference contribution to the LDOS measured by the STM tip, which eventually exhibits oscillations as a function of the magnetic flux. More specifically, the LDOS can be written as N = N₀ + N_loop [cos (πФ/Ф₀) - 1], where N₀ is the LDOS in the absence of the gauge field, N_loop is its interference contribution along the loops, Ф is the magnetic flux through the area enclosed by the loop, and Ф₀ is the flux quantum. This simple setup can be of potential use to detect space variations of the magnetic field at the nanometre scale as discussed in [5].

Figure 1. Sketch of the STM Aharonov-Bohm interferometer proposed in [5]. The STM tip measures the LDOS of a two-dimensional nearly free electron gas formed at the close-packed surface of a noble metal. This LDOS has interference contributions from electrons travelling along the two (clockwise and anticlockwise) scattering paths shown in the figure. The scattering is due to extra atoms adsorbed on the surface, which can be considered as impurities for the 2D electron gas. The gauge A field affects the above interference via the Aharonov-Bohm effect, producing oscillations in the LDOS as described in the text.

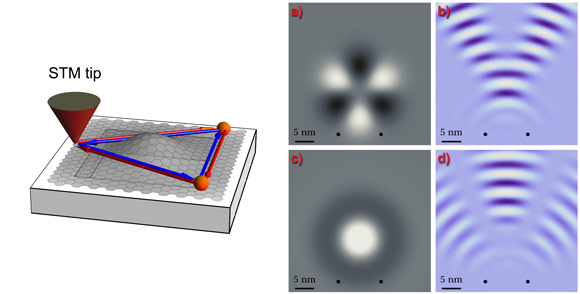
In the case of graphene, electrons are described by an effective Dirac Hamiltonian that can be derived from a microscopic tight-binding model. Then, when the graphene sheet deforms, and the tight-binding parameters change accordingly, the effective Hamiltonian becomes H = - iħv_F σ_i (∂_i -  iA_i), where the effective gauge field A traces back to the change of the electron hopping with the lattice deformations [A  = (κФ₀/π) (u_xx - u_yy, - 2u_xy), with u_ij being the strain tensor] [1]. This brings out the possibility of having Aharonov-Bohm interferences without real magnetic fluxes since, in the case of graphene, having a certain landscape of deformations is analogous of having the system in the presence of a magnetic field. The emergent gauge field A, however, preserves time-reversal symmetry and therefore seems to give no net Aharonov-Bohm effect. In reality, in each of the two different Dirac points, the electrons feel an effective symmetry breaking that is restored only once the system is considered as a whole (because the coupling with the gauge field has opposite sign in the two different Dirac points). As long as the two Dirac points are independent, this suffices to generate Aharonov-Bohm interferences in the LDOS as we have demonstrated in [5]. The result is illustrated in Figure 2, which shows that the above STM setup can also be used to probe tiny deformations of graphene sheet by means corresponding Aharonov-Bohm oscillations.

Figure 2. Left panel: Proposed STM setup for measuring Aharonov-Bohm oscillations due to deformations in graphene. Right panels: Distribution of pseudo magnetic fields generated by (a) out-of-plane Gaussian bump and (c) in-plane displacements with C3 symmetry, and corresponding patterns of Aharonov-Bohm oscillations in the LDOS (b and d respectively). The black dots indicate the position of the scatterers.

Principal publication and authors
F. de Juan (a), A. Cortijo (b), M.A.H. Vozmediano (c) and A. Cano (d), Nature Phys. 7, 810 (2011).
(a) Department of Physics, Indiana University, Bloomington (USA)
(b) Departamento de Física Teórica, Universidad Autónoma de Madrid (Spain)
(c) Instituto de Ciencia de Materiales de Madrid (CSIC) (Spain)
(d) ESRF

References
[1] M.A.H. Vozmediano, M.I. Katsnelson, and F. Guinea, Phys. Rep. 496, 109-148 (2010).
[2] N. Levy et al., Science 329, 544-547 (2010).
[3] Y. Aharonov and D. Bohm, Phys. Rev. 115, 485 (1959).
[4] G. Bergmann, Phys. Rep. 107, 1 (1984).
[5] A. Cano and I. Paul, Phys. Rev. B 80, 153401 (2009).

Top image: An Aharonov-Bohm oscillation pattern.