Giant Kohn anomaly in the low-dimensional chain compound ZrTe3

Low-dimensional materials are especially prone to developing ordered structures beyond the periodicity of the crystal lattice. These structures are only stable at low temperature and a phase transition between the un-ordered high temperature and the ordered low temperature phase is observed. One intriguing example is the incommensurate charge density wave (CDW) where the electrons bunch up according to the wavelength of their wave function. These structures, which can even be incommensurate with the underlying crystal lattice, are observed through the appearance of additional diffraction spots that represent the superstructure when the crystal lattice is statically modulated in the ordered phase. A general theory of the phase transition is mean field theory (MFT). It has been suspected for a long time that MFT should not be applicable to low-dimensional materials, but deviations should be manifest in the lattice dynamics of the material.

A charge density wave is a direct manifestation of the wave nature of electrons in a crystal. Two electrons can never adopt the same pattern of motion, or wavefunction. The consequence is that the electrons arrange themselves in a variety of different wavefunctions with increasing energy until all electrons in the solid have been accommodated. The wavefunctions with the highest energy (the Fermi energy) are easily excited by small thermal energies leading to fluctuations of the charge. In the crystalline solid, each wavefunction corresponds to a propagating motion with a specific wavelength λ. A charge density wave is present if the electrons at the Fermi level bunch up to form a standing wave of their specific wavelength λ_P, which is often not commensurate with the crystal periodicity. This is possible if two conditions are met: there have to be a lot of slightly varying wavefunctions with nearly the same wavelength λ_P at the Fermi level, travelling in the same direction (called Fermi surface nesting) and these electrons have to be easily excitable by the lattice vibrations of the crystal (giving rise to so-called called electron-phonon coupling). The former is easily fulfilled if chain-like arrangements of atoms are found in the solid.

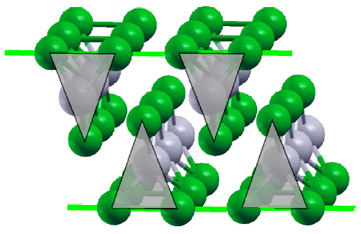
The crystal structure of ZrTe₃ consists of such chains, as shown in Figure 1. The atoms are arranged in alternating Te₃ triangles and Zr ions. These prismatic chains are laterally arranged to form layers. The chain responsible for the charge density wave forms where the triangles are laterally linked and chains of Te atoms are found. Some of the electrons moving along this chain are at the Fermi energy and form the charge density wave.

Figure 1. Crystal structure of ZrTe3.  The Te3 triangles and the Te-Te chains of linked adjacent prisms are indicated.

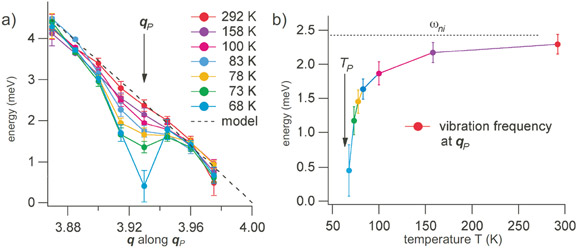
In principle any lattice vibration pattern of wave vector q_P corresponding to the charge density wave could couple to the electrons and be affected by the formation of the standing wave pattern. The first task was thus to identify which vibration mode is affected by the interaction. For this purpose a map of diffuse X-ray scattering was measured just above the transition temperature T_P at the Swiss-Norwegian CRG beamline BM01A by recording the intensity of X-ray scattering between Bragg spots everywhere in reciprocal space. At the reciprocal space position where the strongest effect was found in this survey, the vibration frequencies were measured by inelastic X-ray scattering (IXS) at ID28. This result is shown in Figure 2. A Kohn anomaly in the phonon dispersion was found in the form of a dip at the incommensurate position q_P. This soft mode freezes to zero frequency as the sample is cooled to the transition temperature.The unexpected finding is that the vibration frequency of the soft mode remains quite high over a large temperature range above T_P. Only close to the transition does the frequency drop sharply and then goes to zero, which corresponds to the formation of a static modulation.

Figure 2. a) Phonon dispersion, i.e. vibration frequency as a function of wave number across the charge density wave qP. b) Vibration frequency at qP as a function of temperature down to the transition temperature TP.

This sudden and rapid decay of the vibration frequency has not been observed in other materials, mainly due to the difficulty of performing such an experiment using the classical technique of inelastic neutron scattering. The experiment has become feasible owing to the high brilliance and thus possible fine focusing of synchrotron X-rays. The result obtained by IXS fully supports the important influence of fluctuations in low-dimensional materials.

A second part of the study was devoted to measuring the evolution of the ordering as a function of temperature. The CDW order manifests itself in the development of incommensurate superstructure diffraction spots. Their width in reciprocal space represents the correlation length of the developing charge density wave above the phase transition. The intensity corresponds to the increasing amount of order at and below the phase transition. Close to the transition,  the intensity and the half-width develop with a power law as a function of reduced temperature (T–T_P). The combination of several power law exponents, called critical exponents, allowed the researchers to assign the CDW order in Te₃ to the universality class of a phase transition with an order parameter of dimension n = 2. This class, with its strong deviations from mean field theory, is compatible with the observation of the importance of fluctuations in the vibrational properties of the phase transition.

Principal publication and authors
M. Hoesch (a), A. Bosak (a), D. Chernyshov (b), H. Berger (c), and M. Krisch (a), Giant Kohn anomaly and the phase transition in charge density wave ZrTe3, Phys. Rev. Lett. 102, 086402 (2009).
(a) ESRF
(b) Swiss-Norwegian Beam Lines at the ESRF
(c) Ecole Polytechnique Federale de Lausanne, Institut de Physique de la Matiere Complexe, Lausanne (Switzerland)